Classifying Numbers & Properties (Algebra 2 HN)

This is a classifying numbers and properties review problem set. These questions are coming directly from an algebra 2 quiz, so use it wisely! I really hope this is useful!

1) Convert √(32) into decimal form.

2) Since the decimal form 5.6568... is continuous but NOT repeating, it is irrational.

3) Looking at the classifying numbers chart, all irrational numbers are real. (Memorize the chart.)

4) √(32) is both irrational and real.

1) Natural numbers exclude 0; therefore, 0 isn't natural.

2) Whole numbers include 0; therefore, 0 is a whole number.

3) If 0 is whole, it is also an integer, a rational number, and a real number.

4) 0 is a whole, integer, rational, and real number.

1) 9/3 = 3

2) 3 is a natural number.

3) Natural numbers are whole, integer, rational, and real.

4) 9/3 is a natural, whole, integer, rational, and real number.

1) -12.333... is a decimal; therefore, it wouldn't be natural, whole, or an integer.

2) -12.333... is continuous and repeating, so it would be a rational number.

3) Rational numbers are always real numbers.

4) -12.333... is rational and real.

1) Transitive property:

a=b b=c --> a=c

2) If 5=N And M=5, Then M=N is transitive.

1) Associative property of multiplication.

(a*b)c = (c*b)a

2) (a+7)+9=a(7+9) is the associative property.

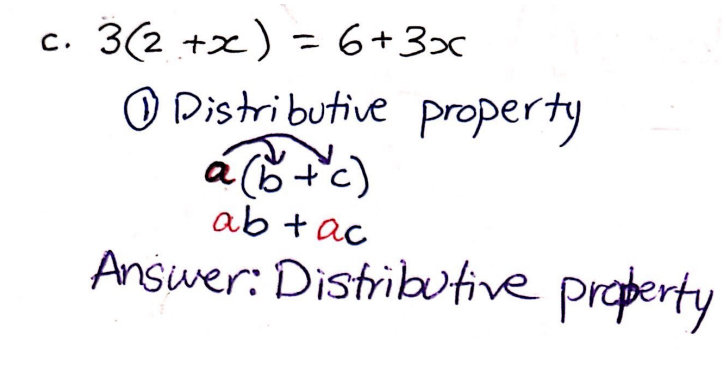
1) Distributive Property

a(b+c) = ab+ac

2) 3(2+x)=6+3x is the distributive property.

1) Additive Inverse Property: The sum of any number and it's inverse always equals to 0.

x + (-x) = 0

2) 5 + (-5)=0 is the Additive Inverse property.

Closed, in mathematical terms, means that 2 numbers of the same classification multiplied, added, subtracted, or divided by each other would equal a number in the same classification.

1) Any 2 rational numbers multiplied will always equal another rational number.

2) Rational Numbers Are Closed Under Multiplication: True

Closed, in mathematical terms, means that 2 numbers of the same classification multiplied, added, subtracted, or divided by each other would equal a number in the same classification.

1) Any 2 whole numbers subtracted from each other will not always equal another whole number.

2) The Whole Numbers Are Closed Under Subtraction: False

Counterexample: 2 - 3 = -1 (integer).